• Information for Parents
• Information for Students
• Information for Educators

The following excerpt is from the new book by Mandee Heller Adler, From Public School to the Ivy League: How to get into a top school without top dollar resources, which is available at Amazon.com. Ms. Adler is the founder and principal of International College Counselors, a Florida-based firm that provides expert strategies for admission to undergraduate colleges, graduate programs, business schools, law schools, medical schools, dental schools and other postgraduate schools.

From Chapter 4: Writing Essays …

ANSWERING THE QUIRKY QUESTIONS
In recent years, a number of colleges have been adding quirky questions to their applications. These supplemental questions are considered a way to get students to stand out from the crowd.

These questions have included:

• Imagine you have to wear a costume for a year of your life. What would you pick and why?
• What is your favorite ride at the amusement park? How does this reflect your approach to life?
• What does Play-Doh have to do with Plato?
• What would you do with a free afternoon tomorrow?
• What was your favorite thing about last Tuesday?
• The Spanish poet Antonio Machado wrote, “Between living and dreaming there is a third thing. Guess it.” Give us your guess.
• According to Henry David Thoreau, “One is not born into the world to do everything, but to do something.” What is your something?

What colleges are looking for is your voice. Use this as an opportunity to demonstrate your “out-of-the-box” thinking. However, don’t go overboard. Admissions officers are looking to see if you’ll be an interesting person to have on campus. Interesting means imaginative, not crazy and not dangerous sounding.

GREAT FIRST SENTENCES
You need a great hook and a great first sentence. Opening sentences have the power to compel and fascinate. Some of our favorite student first sentences include:

• For eight years, I have celebrated polyester.
• I vividly recall coming home from school one day in Buenos Aires, Argentina, to find my house in disarray and my parents packing one suitcase after another.
• I’ll admit it: I have a thing for gavels—a thing for motions and seconds and the clarity that they bring to meetings.
• I eagerly reached into my Hello Kitty backpack.
• Max prances in place as we await our turn into the arena.
• Drip. Drip. Drip. Tick. Tick. Tick. As I lie in the hospital, waiting to be taken into surgery, I can only think that my IV drip sounds just like a metronome.

You want to read more, right?

FATAL ESSAY ERRORS
Application essays have been requested as part of the college application for the past umpteen years. The admissions teams have seen a lot of “creativity.” Here are their least favorite types of essays:

• Metaphor. Don’t compare yourself to a mango, a Ferris wheel, or any other objects.
• Death. Don’t write about a person or pet’s death unless it truly affected your life and you can use it to exemplify growth—for example, if someone died of cancer and you made it your mission to raise money/awareness, or if a death during high school affected your grades and caused you to stumble, but then you regrouped to overcome.
• Free verse essays, essays written as raps, limericks, etc. Don’t emphasize form over function.
• “Meta” essays where you talk about writing an essay, about the process of writing an essay, or about essays themselves.

Additionally, you should avoid writing about the topics below unless you have something extraordinary to say:

• A trip to Europe
• Generic admiration for your mom or dad
• The controversial rock star or movie star whom you idolize
• Overcoming an injury and making an athletic comeback
• Volunteering at a local community center
• Building homes in Costa Rica with Habit for Humanity
• Understanding the meaning of life from a fishing trip

Sorry, but thousands of students have beaten you to these topics and then beaten them to death. These are called “cliché essays” because the reader knows from the get-go just where you are going with it.

THE VIDEO COLLEGE ESSAY
A number of college admissions departments are formally accepting video college essays.

The first step for any student is to view recent videos and see what others have done. This will give you an idea of the range of possibilities.

When it comes to actually making your video, it’s important to be original but in a way that is comfortable for you. Do what works for you. Your main goal needs to be communicating your message.

• Start by identifying the question and any directions.
• Think about what you want to say.
• Write a script that is clear on the message and ideas you want to get across.
• Collect resources and props that you want to use in the video.
• Record the video until it’s as perfect as possible. Some students record the video themselves using a tripod while speaking directly into the camera; others enlist the services of a friend or family member.
• Review your video and collect feedback.
• Edit, edit, edit, and re-record if necessary.
• Get more feedback.
• Edit and re-record until it’s as perfect as it can be. Make sure it fits the requested length and meets all specs before sending it in.

To get a head start on preparing for college admissions, order a copy of From Public School to the Ivy League: How to get into a top school without top dollar resources from Amazon.com.

Broward County, Florida students currently attending grades 6-8 are invited to apply to the IMACS Math Academy, an intensive one-week program designed to stimulate talented students’ interest in mathematics beyond the traditional classroom. There is no cost to attend the IMACS Math Academy!

There are two scheduled sessions. The first will be held March 24 – 28 during Spring Break. The second will be held June 23 – June 27 during Summer Break. Both sessions of the IMACS Math Academy will be held at IMACS Headquarters in Plantation, Florida.

To be considered for one of the two available sessions, students must first complete an online aptitude test by February 28, 2014. Up to 60 students who do well on the aptitude test will be invited to apply to the IMACS Math Academy.

Parents interested in the IMACS Math Academy should visit www.imacs.org/mathacademy.

UPDATE, July 28, 2014: IMACS has completed the update of our AP^® Computer Science: Java Programming course to include eight fully-elaborated labs that far exceed the minimum requirements of the College Board. IMACS’ Be Prepared for the AP^® Computer Science Exam online course has been updated as well. Students who are enrolled directly through eIMACS in our AP^® Computer Science: Java Programming online course receive free access to the Be Prepared course.

Following a recent review of the AP^® Computer Science A course and exam, the College Board has decided to replace its case study requirement with a requirement to complete a minimum of 20 hours of hands-on lab experiences. This change, which will take effect for the 2014-2015 school year, is being implemented to more effectively support student learning of core concepts in computer science. IMACS continues to follow closely all communications from the College Board, as well as discussions within the APCS community, on the forthcoming changes and will act accordingly.

From the beginning, IMACS’s philosophy has been to emphasize computational thinking and mastery of foundational ideas in computer science. This approach is reflected in how our Curriculum Development Group has meticulously designed our CS courses and, more importantly, in the success our CS graduates find in college, graduate school and at top tech companies. As such, IMACS fully expects that our AP^® Computer Science: Java Programming course will continue to exceed, as it always has, all of the College Board’s requirements and remain College Board-approved.

GridWorld Case Study

Since the 2007-2008 school year, AP^® Computer Science A has used the GridWorld Case Study to reinforce lessons on object-oriented programming.* GridWorld provides Java code designed to simulate the behavior of objects (Rock, Flower, Bug and Critter) in a grid. Ground rules such as Rocks cannot move, Critters eat Flowers and Bugs move forward and turn 45 degrees if blocked are part of the initial set-up. Given these starting parameters, students then write additional code that extends these various classes of objects. A student’s understanding of computer science concepts in the context of the GridWorld code is then tested on the AP exam with one free-response question and a handful of multiple choice questions.

College Curriculum Study

In 2011, the College Board undertook a College Curriculum Study in which institutions of higher education were surveyed about the AP^® Computer Science A course case study.^ Of the 117 institutions that responded, 91% said they were not likely to change their credit/placement policy for AP^® CS A if questions on the case study were not included in the exam. About two-thirds of respondents rated the inclusion of a case study as not important or only somewhat important.

Clearly, GridWorld is now past its prime. As the College Board noted on its website, the case study requirement in AP^® Computer Science A needed updating “to stay aligned with the most recent practices in the continually changing field of computer science.”

Labs, Labs, and More Labs

This March, the College Board plans to release details of three sample AP^® Computer Science A labs as examples of how the new lab experience requirement may be implemented. One expectation is that their shorter length will make the labs easier to integrate into the course curriculum throughout the school year. Teachers and curriculum developers will have the flexibility to include sample labs or other comparable labs at points they feel are most relevant and pedagogically effective. It is also expected that the sample labs will be more connected to real-world situations, perhaps increasing student interest in taking the course and studying computer science.

Most importantly, labs are expected to support student learning of fundamental ideas in computer science. Whereas the case study questions on the current exam are tied heavily to the context of the GridWorld code, the 2015 AP^® Computer Science A Exam will test a student’s understanding of core concepts that are reinforced by hands-on lab experience, not knowledge specific to any particular lab. As an educational institution that has always emphasized foundational concepts in CS over code manipulation skills in the programming language du jour, IMACS is pleased to see the College Board take this important step.

Learn how you can give your child an unfair advantage in computer science. To find an IMACS teaching center near you, visit www.imacs.org. Talented middle and high school students can take university-level computer science online through our eIMACS distance-learning division.

*For readers who may be unfamiliar with object-oriented programming, it’s an approach in which the programmer creates “objects” with specified attributes and behaviors as modular, reusable code.
^https://tinyurl.com/LabsAPAC2013

The following letter is extracted from the new book, Letters to a Young Math Teacher, by Gerald Rising and Ray Patenaude, which is available from Amazon.com and other sources. Gerry Rising is Distinguished Teaching Professor Emeritus at State University of New York at Buffalo where he co-founded the university’s Gifted Math Program for highly-qualified regional students in grades seven through twelve. Gerry Rising was for many years associated with IMACS activities and is a strong supporter of our work.

Paul Rosenbloom enjoyed an international reputation as a senior mathematician when I joined his Minnemath Project at the University of Minnesota as his assistant director. His Elements of Mathematical Logic remains today a basic resource recognized worldwide to be of historical as well as academic significance.

In our very first conversation he told me that he considered teaching outside the classroom an important aspect of a mathematician’s life and he urged me to develop what he called “a bag of tricks” from which to draw math-related lessons for people of all ages. I would later see him draw from his own collection in a number of settings. He could captivate anyone from nursery school students to scientific colleagues and even senior political figures.

Within a month of that conversation I found myself seated on an airplane flying from Minneapolis to Denver. My seat partners were a bored nine-year-old and her mother. When the mother learned that I was a math teacher, she asked me if I would be willing to “show Marjorie some math.”

Fortunately, I had been reading David Silverman’s book, Your Move, and I had some pennies with me. I played a series of Nim games with Marjorie, finding her an interested opponent and a remarkably quick study.

Nim, for readers unfamiliar with the word, is German for “take” and in this context it applies to games with players taking one or more counters like coins from a collection following a given set of rules.

We started with One Pile Nim. I set out a line of pennies on Marjorie’s tray and explained the rules. We would take turns, at each turn removing one, two or three coins. The winner would be the player who could take the last penny or pennies.* When she quickly determined the strategy for playing, I suggested she challenge her mother. Her eyes lit up as she outplayed her mom.

Now she wanted more. I suggested the misère form of the game: same rules for play but this time the loser forced to take the last coin. Now I had her mother’s full attention as well, but again Marjorie, once she found the winning strategy, could outplay mom.

We went on to play the very simple Two Pile Nim game in which each player takes any number of coins from one of two piles with the winner taking the last coin, but then the less transparent game, Woolworth, which is isomorphic to Two Pile Nim. Woolworth is named for the five-and-ten-cent stores of the 1950s that have been reincarnated as today’s Dollar Stores. On a sheet of paper I drew the following diagram:

I placed nickels and dimes as shown in the figure. One player controls the coins on the left, the other the coins on the right. Players alternate moving either of their coins any number of squares to left or right but without joining or passing the opponent’s coins. The object of the game is to squeeze your opponent so that no moves remain.

By the time I showed Marjorie the connection between Two Pile Nim and Woolworth we were well on our descent into the Denver airport. I was rewarded for our hour together when Marjorie turned to her mother to tell her that she wanted to become a mathematician.

Where do you find lessons like these that require no background and yet are suitable to given age groups? I found mine from two sources: my reading of journals and books about mathematics and my observation of lessons taught by others. Appendix 2 lists a few of the many available book sources.

Now, because it is a good story, I will tell you about my use of a lesson copied from the remarkable teacher, Robert Wirtz.^

Late one school year when I was a math supervisor I was asked by a primary school teacher to demonstrate Stern blocks for her students. Stern blocks are inch cross-section blocks that come in lengths from one to ten inches. Cuisenaire rods are similar but with centimeter dimensions.

I took several sets of Stern blocks to this teacher’s classroom and showed the six-year-olds some of the relationships among them.

The students were excited by the opportunity to mix play with learning, and I was convinced that they were gaining from the lesson. I noticed, however, that their teacher was not happy with the children’s mix of play with learning. Several times she interrupted their activities to tell individuals to be quiet.

It was clear to me that the teacher was convinced that I wasn’t disciplining the children in the way she wished, so I suggested that I return the next day to teach another lesson. Despite her reservations about my conduct of this class, she jumped at the chance to have me return. It was clear that she was happy to pass responsibility for these irrepressible kids off onto anyone she could find.

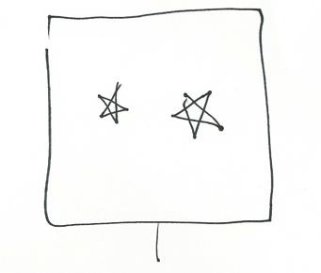
I had seen Bob teach a lesson to a similar group and the next day followed his model. Before the class I had the teacher gather the students in front of the chalkboard. Saying nothing to them I drew a square on the board and within it marked two star shapes. What I had drawn looked like this:

I pointed to that little tail at the bottom of the figure and turned to the class, holding out the chalk.

Several hands waved and I offered the chalk to one of the wavers. The boy came up and carefully wrote a “2” below the diagram.

That got us started. I drew similar diagrams with different shapes and the children quickly caught on, competing to write the numbers below the figures. Then I began to complicate the requests by erasing their numbers and connecting two figures like this:

No problem. A volunteer wrote “5” below the boxes.

I continued in this fashion, soon replacing the boxes with numbers but using the same connecting segments. All of the children were eagerly participating, but not one word was said by me or by any of them.

After a half hour of this activity, I finally spoke up, calling the children’s attention to the fact that none of us had spoken until then. And I turned to their teacher to congratulate them on what we had accomplished.

Unfortunately, my lesson proved nothing to this young woman. Her response: “Yes, after you left yesterday I really scolded them for their behavior and you saw the result this morning.”

Even with the best of efforts, you cannot always win.

I have talked about these mathematical extras mostly in terms of their use outside of your classroom, but they can play a role in your classes as well. Here is an example described by Swedish math educator Barbro Grevholm:

Please take the examples I have used only as samples of my own and others’ collections of activities. You may or may not add them to your collection. What is important is that you accumulate such activities that work for you. They will serve you well.

Sources of such activities are all around you. You just have to be alert to them and adapt them creatively.

Many such activities come from your reading. Mathematics and mathematics education journals describe topics and lessons that you can both enjoy and share with others. Of course, you should credit your source when you replicate such a lesson, but every writer I know would be delighted to know that his or her lesson was being duplicated.

The two best journal sources I know for such examples are the Mathematical Association of America’s Math Horizons and the British Mathematical Gazette.

Some non-standard texts are full of such lessons. Authors like Harold Jacobs and Sherman Stein are two who have gathered and present such useful topics. And some authors simply collect such presentations. Among these are Ian Stewart, Ross Honsberger and Howard Eves.

In a class by himself is Martin Gardner whose collections of such topics are unsurpassed. His range is amazing, covering everything from hexaflexagons to fractal music, almost all of them serving a perfect basis for well-planned demonstrations.

Even your daily newspaper can provide activities. Although many people are drawn to the Sudoku puzzles, I much prefer three other popular forms: Kakuro, KenKen and Numbrix. These puzzels are adaptable to students of all ages (I solve one or two each day) and can challenge them all. The simplest appearing is Marilyn vos Savant’s Numbrix. While this puzzle task is appropriate for primary grade students (all it involves is listing the integers from 1 to 81 in order in a 9×9 grid), individual Numbrix puzzles range widely in difficulty.

In addition to their intellectual challenge, there are two things about the Kakuro and KenKen puzzles that I find attractive: (1) they involve basic calculation facts that reinforce the solver’s skills and (2) they appear as free apps on mobile phones and tablets. This availability makes them perfect puzzles to introduce to fellow travelers.

* The strategies for playing these Nim games are included in Appendix 4. I encourage you to play the games before you look at those strategies.

^ Bob Wirtz and his wife adopted a number of children with severe learning problems and they developed math materials to teach them. With Mark Botel, then president of the International Reading Association, he gathered those materials into books for elementary school students. Sadly, they are difficult to find today for they include some very attractive activities.

Abstract reasoning ability entered the national conversation this year as the Common Core State Standards in mathematics were broadly implemented in the United States. In particular, one of the eight Standards for Mathematical Practice is to “reason abstractly and quantitatively.” The so-called STEM subjects — science, technology, engineering and math — are well-known for emphasizing this skill. Given that STEM-related fields are where most high-skilled job growth is predicted, today’s students would do well to develop their ability to think abstractly.

So what is abstract reasoning, and why is it so important? Let’s break it down: To reason is to use logic in piecing together information, usually with the goal of forming an inference or conclusion. Abstract simply means that this process is a thought-based exercise of the mind as opposed to being based in concrete experience. For example, if you know that ice melts at temperatures above 32°F, you can reason abstractly that an ice cube placed on the counter of your room temperature kitchen will melt. You don’t have to take an actual ice cube out of your freezer and observe it for an hour to arrive at this conclusion.

Of the subjects that you could study in order to develop strong abstract reasoning skills, computer science is a natural and practical choice, as well as being a highly creative and exciting area in which to learn and work. The programming aspect of computer science is well-known and is one area where abstract thinking matters a great deal. Programming, after all, is the creation of a set of instructions that a computer can follow to perform a specific task. Such tasks typically involve the manipulation of digital information, decidedly not the kind of stuff you can grab hold of to see how it reacts in the tangible world.

Learning to program well involves developing the ability to think logically and abstractly so that you can anticipate how the computer will react to the instructions you give. Great programmers are actually capable of writing simple code without having to check it with a computer because they have the ability to analyze processes in their minds. If you cannot think abstractly, you may still be able to get your code to “work” with trial-and-error tinkering, but that approach lacks the robustness needed to solve meaningful problems that tend to be more complex.

The rich experience of learning computer science, however, is so much more than coding. When you study computer science, you engage in computational thinking, in which logic, abstraction and creativity come together to help solve intellectually interesting problems. As Professor Jeannette Wing of Carnegie Mellon University argues in her seminal article* on the topic, computational thinking is a skill set from which everyone would benefit no matter their career path.

Why so? Because when you study computer science, your mind learns to grapple with high-level questions such as: How can existing information be used to deduce further information that will help solve the problem? How should a complex system be designed in order to maximize simplicity and usability? How can a complex problem be broken down into smaller pieces that are easier to solve? Can a common approach be devised to efficiently handle similar problems?

If these questions seem like they would be applicable in a wide variety of fields, STEM and non-STEM, it’s because they are. In essence, when you study computer science you learn the valuable skill of thinking abstractly like a computer scientist even if you don’t plan on becoming one.

*Wing, Jeannette M. “Computational Thinking.” Communications of the ACM 49:3 (March 2006) 33-35.

IMACS hopes this Thanksgiving day finds you with much to be thankful for as you enjoy the company of friends and loved ones. To our students, parents, instructors, staff and partner schools — you have our deepest appreciation for making this another fun-filled year of learning and achievement!

The IMACS Blog will return next Thursday, December 5, with a regular feature article. Happy Thanksgiving!

A recent study found that number sense in infants is a predictor of how well they later learn the symbolic math typically taught in school. Researchers observed how long 6-month-old infants looked at a screen with a changing number of dots compared with how long they looked at a screen with a constant number of dots in changing patterns. (See video above.) Three years later, these children were given an IQ test and several types of math tests. The infants who looked longer at the screen with a changing number of dots, which is indicative of stronger number sense, subsequently scored higher on the math tests, regardless of IQ. So what is "number sense," and does this mean that parents should start packing dotted flash cards in the diaper bag?

What is Number Sense?
There are different definitions of number sense, but they generally agree on a few basic factors:

• Number sense is intuitive. In other words it is not arrived at through conscious reasoning.

• Number sense includes an understanding of magnitude of amounts, relationships between amounts, and how amounts change through natural actions.

Examples of number sense in an infant might include observing and understanding the following: There are a lot of stars on my blanket. Daddy has more food on his plate than Mommy. I have a bunch of blocks on my high chair table. If I knock some off, I have fewer blocks. From what current science tells us, pre-verbal infants cannot actually have these words running through their heads. Nonetheless, a primitive understanding is there as humans seem to be born with an innate ability to perceive quantity before they can speak about it and well before they can write about it.

Nurturing Number Sense
So should you start showing your baby flash cards with dots? No. While the study’s authors hope that their findings will lead to improved strategies for math education, they are careful to caution that "[O]ur infant task only explains a small percentage of the variance in young children’s math performance. But our findings suggest that there is cognitive overlap between primitive number sense and symbolic math. These are fundamental building blocks."

Still, we know that there are benefits to talking to your baby as an essential part of the early learning process. It’s not unusual to hear a mother carrying on a one-sided conversation in which she emphasizes the phonetic sounds in the names of everyday objects. For example, "This is a banana. Buh buh banana." The baby eventually catches on that the "buh" sound is associated with a banana, and that Mom’s lips do this strange "roll in-then-pop out" motion when she makes this sound. This happens before the baby ever learns to say "banana" or that there is this symbol we call the letter "b" that represents this sound. When the letter "b" is finally introduced, children can connect it back to the "buh" sound and objects such as a banana to which they were introduced in infancy.

But how frequently do you notice a parent holding up two different bananas and talk about which one is bigger, or which one has more brown dots? Or what happens to a bunch of bananas when you pull one off? Or when you cut that banana into pieces? The study’s lead author indicates that "We believe that when children learn the meaning of number words and symbols, they’re likely mapping those meanings onto pre-verbal representations of number that they already have in infancy." If she is correct then adding number sense talk to those parent-baby conversations makes, well, sense!

The new school year is now a month old. By this time, most children who attend a public K-12 school in the US will have experienced the new Common Core State Standards in Mathematics (CCSSM). On the one hand, IMACS is pleased to see that key elements of the teaching philosophy we have lived by for more than 20 years are reflected in the Common Core Standards for Mathematical Practice. For a variety of reasons, however, we maintain a healthy amount of skepticism about whether the implementation of the CCSSM will lead to meaningful, positive change in mathematics education, particularly for our most talented youth.

Common Core Was Not Designed for Gifted Kids

First, the CCSSM was not designed with exceptional kids in mind. The official Common Core Web site states plainly that:

The Web site further acknowledges that Common Core, like its predecessors, cannot adequately address the unique needs of individual learners:

As to what educators should do about serving the diverse needs of a student body, Common Core guidance leaves them with unresolved internal conflict, offering both:

and

[Note that Common Core does not include gifted children as an example of “students with special education needs.”]

But children in the right-hand tail of the distribution do have special education needs. Whether due to a failure to understand this fact, budgetary pressure, or some other constraint, some school districts seem to be latching on to (ii) above, using the arrival of Common Core as a reason to reduce or eliminate services or accommodations for gifted students. Should this become a national trend in education policy, our country will surely suffer as the majority of gifted children who rely on public education are left without appropriate alternatives.

What About Creative Problem Solvers?

Notwithstanding the potential for improving the thinking skills of typical students, the CCSSM are simply not built to inspire or nurture the creative problem solver. The unfortunate embracing of computerized testing as a cheap means of measuring “learning” — consequently resulting in a culture of teaching to the test — has made the K-12 classroom a place to dread for many unique thinkers. The plan to continue use of computerized testing under the new standards suggests that the non-standard thinker may still be out of place in the Common Core classroom.

IMACS recently asked Gerald R. Rising, SUNY Distinguished Teaching Professor Emeritus at the University at Buffalo, how he thought the CCSSM would affect mathematics education for bright children, to which he replied, “Any imposed curriculum can have a depressing effect on special programs for gifted students.” He also shared the following anecdote about the limits of standardized testing:

IMACS has been delivering courses and administering tests online to bright and creative children for over 15 years, so we know a thing or two about designing effective computerized assessments of high-level thinking skills. Let’s just say that it takes tremendous creativity, foresight, and a deep understanding of how to leverage the power of technology. If high-stakes testing is here to stay, as it appears to be, we sincerely hope that the consortia working on Common Core-aligned assessments will find ways to reward (or at least not penalize) creative problem solvers.

Inadequate Investment in Training

Common Core marks a major change in teaching philosophy for math education in the US. The intent is to move away from just teaching procedural skills by giving equal weight to conceptual understanding. Teaching math with an emphasis on thinking and understanding, however, is not something one becomes proficient in after a few hours of training, which is all that many districts have provided to their teachers.

Such a radical shift in mindset can be especially challenging for some who have taught math with a completely different focus for many, many years. This is not to say that teachers are incapable of learning to teach a new way — quite the contrary. But, as with any field undergoing fundamental change, extensive training and professional development are necessary if districts and schools want a successful implementation of Common Core. So far, the evidence suggests that they cannot or will not be making that investment.

“Common Core” Textbooks In Name Only

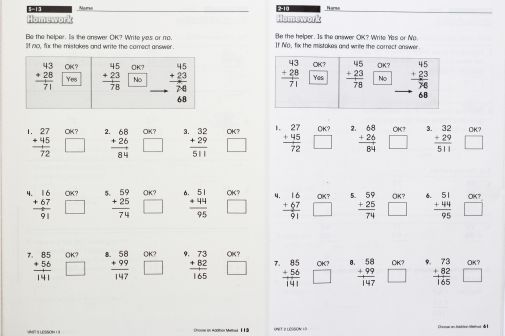
Many of the textbooks currently on the market that say they are aligned to the Common Core standards were developed before the creation of the CCSSM. Note the example below of pages from old and new versions of a math text currently being used in California. The pages on the left were from the edition published in 2009, the year before states began adopting the CCSSM. The pages on the right are from the current edition that proclaims “Common Core” on the cover. (Click on an image to enlarge.)

Furthermore, such textbooks often only align to the specific content skills listed in the CCSSM rather than subscribing to the overall philosophy of the CCSSM. Many that claim to be aligned to the CCSSM do not include problems or tasks that involve the higher-level thinking skills that are supposed to be measured by the new Common Core standardized tests being developed.

Awareness and Advocacy Are More Important Now Than Ever

What does all this mean if you are the parent of a talented child? Probably more work for you. Just over a month ago, nearly two-thirds of respondents to a poll on education said they had never even heard of Common Core! So, if you’re thinking that someone else will speak up first, don’t count on it. Advocacy for a gifted student has never been easy given the lack of awareness and amount of misinformation about their unique educational needs. With the potential for Common Core to bring more harm than good to the education of exceptionally bright kids, it is more important now than ever to be heard.

This month the IMACS Blog speaks with IMACS student, Fiona Brady. According to Fiona’s mom, Susan, “IMACS was the first time Fiona had encountered a community of teachers and learners who were excited to hear her ideas and creative ways of problem solving.” After the Brady family moved out of the area, Fiona continued taking courses through our distance-learning program, eIMACS.

Having studied University Computer Science and AP® Computer Science through eIMACS (and scoring a 5 on the AP® exam), Fiona was able to pick up the Python programming language* when she encountered it at a summer mathematics camp at the University of Chicago with students several years older than she.

For students as talented as Fiona, homeschooling and early college courses often make the most sense as they and their families seek educational options that provide enough challenge, flexibility and inspiration to help them reach their highest potential. Let’s hear what Fiona has to say about pursuing this path:

Please tell our readers a little about yourself.

I’m turning sixteen this fall and I’m in tenth grade. I’m a second degree black belt in Tae Kwon Do, and I enjoy figure skating and horseback riding. I don’t feel like this gives a real image of me, but there it is. I enjoy making things with cardboard and duct tape, but definitely not wallets. I’ve made my Halloween costumes for the last few years. The year before last, I was Medusa. I wore a snake hat that I built in my bedroom and needed to turn sideways to get out. I have since learned that on some occasions it is important to get dressed outside of your room. When I’m not doing math, I love reading.

You are homeschooled and also taking college classes at Northwestern University. What is it like to do both? How do you balance the academic workload, extracurriculars and time with friends and family?

Homeschooling is not like regular school because there is no large division between having fun and learning. So I don’t balance it. However, when I have a large assignment due, my mom probably doesn’t see me for two days. My extracurricular activities — skating, horseback riding and Tae Kwon Do — force me to do something active. I also enjoy volunteering at the barn where I ride because they work with children with special needs. Our three dogs keep me pretty busy too, especially my own puppy, Mole (named because the white fur around his nose made him resemble a star-nosed mole when I first got him).

What circumstances led you to take university classes?

I have always liked math, so I started taking more than one math class a year. In my eighth grade year I took five. After that, I sort of ran out of other options. I participate in the University of Chicago’s Young Scholars Program, which is led by Professor Paul Sally. He and others at Chicago gave me advice and helped to set up a meeting with the head of the Northwestern Math Department, Professor Mike Stein. Professor Stein gave me permission to sit in on the courses, and introduced me to the professors.

Which classes are you taking at Northwestern? How did your IMACS courses prepare you for those classes?

Last year I took a course on Abstract Algebra and one on Multivariable Calculus and Linear Algebra. This year I am taking Physics and Analysis. IMACS was the first place where I encountered the idea that to learn something you have to own it; that is, you have to be able to form a picture of it in your head, and you need to be able to construct it from basic principles. In the IMACS computer science classes I took, you really needed to do that, otherwise you would get lost in the middle of writing a program and forget what you were doing. IMACS Logic for Mathematics is a continuation of that because it is constructing the basic principles of mathematics, which are skipped over in most high school classes (but assumed to be known in college courses).

[Editor’s Note: Three years running, Fiona has received the award from the Northwestern Mathematics Department for outstanding achievement in mathematics by a high school student.]

What advice would you give to young students who are thinking about taking university classes before they officially enter college?

Ask your teacher questions. I’ve had people in classes ask me questions, instead of asking the teacher. That’s a really big mistake, and it’s an even worse mistake to make in college because the professors are amazing. One of the things I most admire about the professors I’ve had at Northwestern is the unshakably solid understanding they have of the material. Also, if your professor asks the class a question and you think you know the answer, you should raise your hand. Even if your answer is not correct, that just gives you the opportunity to ask a question and figure out what you don’t understand before you try to learn something that builds on it or have a test.

What do you see yourself doing in the future?

I have three more years before I go to college and I want to keep taking classes and learning more. Being a professor sounds like an interesting career. (Being a stuntman does, too, but I don’t think I’ll pursue that.)

*IMACS added Python to University Computer Science II in November 2012 after Fiona had completed the course.

UPDATE, May 27, 2014: IMACS student, Peyton Robertson, impressed President Barack Obama at today’s fourth annual White House Science Fair. Watch Peyton with President Obama starting around the 4:35 mark of this video.

UPDATE, October 9, 2013: Congratulations to IMACS student, Peyton Robertson, on winning the title of “America’s Top Young Scientist,” $25,000 and a trip to Costa Rica! Watch Peyton’s winning moment.

From the moment you meet IMACS student, Peyton Robertson, you can’t help feeling that he is one of those bright, young people who is going to leave his mark on the world in a big way. His creative ideas and energy seem boundless. His smile and enthusiasm are infectious. And he’s just 11 years old!

Most recently, Peyton entered the 2013 Discovery Education 3M Young Scientist Challenge and is one of only 10 finalists (and the youngest) in this prestigious national science competition for 5th through 8th graders. The Young Scientist Challenge encourages students to explore science and innovation during the pre-teen years when interest in math and science typically starts to decline.

Peyton’s project, SOS: Sandless Operational Sandbags, focuses on developing a more effective and less costly sandbag design to protect against damage from saltwater flooding. As Peyton notes in his finalist video, 80% of the $43 billion dollars worth of flood damage caused since 2005 has been from saltwater flooding. Peyton’s native Florida is at risk for hurricane-driven saltwater flooding every year. So he’s decided to do something about it by re-engineering the basic tool of flood control: the sandbag.

The design of traditional sand-filled sandbags means that they are heavy, difficult to transport and, when stacked, leave gaps through which water readily flows. Peyton’s design uses a thin, expandable polymer to keep his bags light and easy to transport. He also pre-fills his bags with enough salt so that when it dissolves, the salt content of the solution inside is higher than that of seawater, helping to keep the seawater from penetrating the bags. Finally, Peyton uses an ingenious interlocking design to minimize the gaps between the bags when they swell. When the water recedes and the bags dry out, they return to their thin, easily transported and stored form, ready for use during the next storm.

IMACS is so proud of Peyton and delighted to count him as one of our many exceptional students. When we asked Peyton’s mom, Shannon Robertson, to describe the influence that IMACS has had on his education, this is what she had to say:

Congratulations, Peyton! Your friends at IMACS wish you the best in the finals of the 2013 Young Scientist Challenge.