Acceleration Helps But Gifted Kids Need More
For mathematically talented children, acceleration is a commonly used approach to address their need to be challenged. While acceleration can save gifted math students from unnecessary repetition, by definition acceleration cannot go deeper than the standard curriculum, and deeper is what many of these students desperately need. Mathematically talented students deserve to be inspired by engaging curriculum, not merely less bored. Educators know this and are seeking better solutions that offer gifted math students the opportunity to study genuine mathematics with the depth and sophistication that match how their gifted minds work.
Common Characteristics of Gifted Children
The National Association for Gifted Children (NAGC) lists common characteristics of gifted children. Twelve that relate particularly to the study of mathematics are:
- Rapid learner; puts thoughts together quickly *
- Excellent memory *
- Advanced comprehension of abstract ideas
- Enjoys solving problems, especially with numbers and puzzles
- Thinking is abstract, complex, logical, and insightful
- Longer attention span and intense concentration
- Learn basic skills quickly and with little practice *
- Asks probing questions
- Highly developed curiosity
- Interest in experimenting and doing things differently
- Puts idea or things together that are not typical
- Vivid imaginations
Of these twelve characteristics, acceleration can make maximum use out of only 1, 2 and 7 to deliver a learning experience that is somewhat better than no accommodation at all. The other nine characteristics require an approach that is fundamentally different from the standard curriculum, which is designed to work with typical students.
EMF Understands Gifted Thinkers
Elements of Mathematics: Foundations (EMF) provides that fundamentally different approach. Developed by a team of mathematicians who teach gifted children, EMF was designed from scratch to leverage all 12 characteristics. The result is a curriculum that allows talented students to immerse themselves in the study of mathematics where their unique way of thinking fits naturally and is an enormous advantage to learning at the highest levels.
One of the most important aspects of EMF is that it allows students to experience the excitement and satisfaction of intellectual discovery. The standard curriculum, accelerated or not, tends to present the final result as a given to be accepted blindly. By contrast EMF emphasizes the path leading up to the result and teaches some basic techniques of logic used to get there.
EMF students are guided through thought-provoking exercises that lead them to keen observations. Then using logical and creative thinking to analyze and synthesize this information, students are able to arrive at the result themselves. This approach creates a much deeper, intuitive understanding of mathematics and is strongly aligned with how gifted children learn, especially the nine characteristics that acceleration ignores.
Gifted Math 2.0
Acceleration has been an acceptable compromise solution for gifted math students and their educators for a long time. Many gifted students, however, have long needed and deserve better. They need to learn mathematics in a way that embraces their endless curiosity and unique way of looking at the world. They need a curriculum designed specifically for the way their minds work. That curriculum is EMF.
IMACS Staff Writer @ 1:00 am
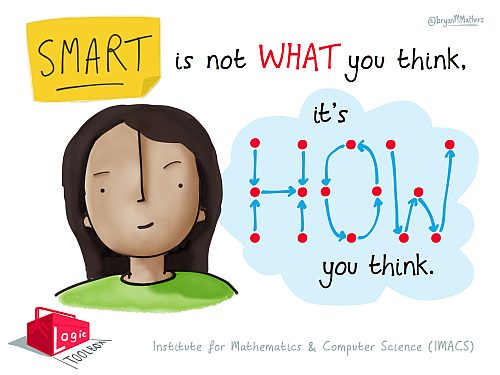
There was a time when the ability to faithfully recall information was a strong determinant of success. Now we have highly-sophisticated search engines and revolutionary chatbots that speak answers to your spoken questions. What you know and think is no longer the definitive measure of being smart. What matters now and in the future is how you make use of the vast knowledge that is readily available. The modern measure of being smart is in how you think.
Leverage and Extend Existing Knowledge
When it comes to knowledge, success today is more about whether you can combine and extend what is already known in order to address novel situations. For example, competitive business environments shift more rapidly than in the past. What worked yesterday no longer works today. As a result, companies have less need for employees to retrieve information or repeat past processes. Instead they have a growing need for people who can reconfigure knowledge to solve problems in real time. Being an encyclopedia of knowledge might make you a Jeopardy! champion, but in the information economy, if that is all you have to offer, it makes you obsolete.
Of course, you need to have some existing knowledge before you can extend it. But talented, young learners can often master the "what" quickly and thus need earlier and more frequent opportunities to explore deeply the "how" and "why". Parents and teachers can support these children by minimizing repetitive work and shifting learning toward activities that ask students to use what they know rather than show what they know.
There is still a meaningful way that existing knowledge by itself can help to develop thinking skills — by understanding how the knowledge came to be. In most educational settings knowledge is delivered as fully formed and tidy, which bears no resemblance to the reality of the non-linear struggles and strides that define the paths to new discoveries. Research shows that understanding the challenges that famous scientists faced motivates students to put forth greater effort on hard problems than merely knowing about the scientists' accomplishments. Another study focusing on teachers' knowledge of math history suggests that teachers who understand that mathematics is an active and creative field can help their students see math as a place for inventive problem-solving where questioning and investigating are highly valued.
Solve Problems but also Learn to Spot Them
Many education-focused organizations emphasize problem-solving skills. Such skills can be thought of on three broad levels. The entry level is to solve problems that are given to you with conventional approaches that are taught to you. At this basic level, success is a matter of how well you reproduce the steps that you have memorized. But as with knowledge, being smart today is about more than this simple kind of problem-solving.
The next level is to solve problems that are given to you with approaches that you try to construct yourself. This level requires a more sophisticated way of thinking because you will need to build on what you already know to come up with approaches that are new to you. To develop these kinds of problem-solving skills, you need more than your existing knowledge; you need analytical tools to make use of that knowledge. For example, you need to be able to see inside the larger problem to its component parts, match up what you know to these smaller problems, and then glue the solution together. Developing problem-solving skills at this level is an extended process best started when children are young. But doing so is well worth the investment because without this foundation, children cannot move on to the next level.
At the most sophisticated level, problem-solving starts with being able to identify problems and ask questions that are less apparent to others. Problem-spotting pushes thinking to the next level because it requires a critical eye toward the conventional and a forward-thinking mind about what the future will bring. This is the kind of thinking that is highly valued in university life and in a fast-paced, global economy because it is the engine for innovative ideas that move humanity forwards. Parents and teachers can plant the seeds for this highest level of problem-solving by creating an intellectual environment in which young people feel safe in both challenging conventional thinking and in having their thinking challenged.
Bright, young learners want more than to merely know what is known and do what has already been done. Satisfying their intellectual curiosity must include opportunities to do more than demonstrate mastery of existing knowledge and procedures. Talented students want to be smart by today's standards. Parents and teachers can support them by providing educational opportunities that leverage their knowledge and stretch their problem-solving skills.
As parents we instinctively want to help our children when they struggle. Our impulse is often to just fix the problem ourselves. While this approach makes sense when children are very young, as they grow it becomes counterproductive. In rescuing our kids, we provide a quick fix but deny them opportunities to practice important skills that they will need to become independent adults.
This is true in general and certainly with respect to education. As children grow, we should encourage them to develop the thinking skills that they will need in college and in their chosen careers. In what may seem like counterintuitive advice, teaching kids to think sometimes involves parents doing less, not more. Here are five tips on how parents can help children learn to think for themselves.
1. Don't be so quick to offer help. If your child asks you for help with school work, first consider how much independent effort she has truly given before turning to you. In a world of online programs that provide hints at the click of a button, it's easy to fall into the trap of thinking that getting to the answer quickly is good and struggling is bad. But math education research shows that a healthy amount of struggle actually leads to deeper learning. By answering your child's questions too soon, you can adversely affect how well she learns. Instead, remind her that it is perfectly normal to take a good amount time to work through problems, allow sufficient time in her schedule to do so, and encourage her to be patient with herself. Once you sense that she has put in a reasonable amount of independent effort on a problem, it's okay to offer hints that give just enough information to get her thinking again without giving away too much.
2. Don't point out mistakes before children have had a chance to discover the mistakes themselves. When you point out mistakes to your child too soon, he is less likely check his own work because he knows you'll do it for him. You also curtail the learning and brain growth that happens when he has to determine on his own that he made an error and what the error is. Finally, you may be signaling that you disapprove of making mistakes, which can lead to other problems such as an unwillingness to try challenging activities. When it's time to bring mistakes to your child's attention, a better way is to let him know that he has made at least one error but not tell him what, where, or how many. This approach necessitates that he will have to look carefully over all of his work and encourages the good habit of doing so on his own as he gets older.
3. Do encourage children to correct their mistakes. Too often, bright students just want to forget about the mistakes they make on school work, especially if they have been led to believe that smart people don't make mistakes. But a mistake means that the problem was challenging for your child, so she should definitely look back at it and work to correct her understanding. Often times we learn better from mistakes than from successes, and research shows that our brains actually grow more from making and correcting mistakes. Help your child see the good in mistakes by using them as opportunities to learn more.
4. Do ask open-ended questions. Asking questions is a highly effective way of guiding your child to think his own way to the answer — What information do you have? What information are you missing that could be helpful? How can you break up the problem into more manageable pieces? When your child was young, he probably asked you a lot of "Why?" questions. As he gets older, turn the tables and ask him why in the same inquisitive, non-judgmental way. Why do you make this assumption? Why do you take that approach? Why does this lead to that? As you know from being on the answering end of "Why?" questions, formulating a thoughtful response takes effort. For a growing mind, that's a good thing. One caveat: Be sure to avoid doing the thinking for your child by giving away too much information in the form of a question such as, "Shouldn't you combine like terms first?"
5. Do provide challenges that require deep thinking. Just as your muscles won't grow from lifting light weights, your child's thinking skills won't develop from doing easy problems. What's more, curious, young minds are naturally engaged by interesting, hard problems as long as those minds are not yet overcome with a fear of making mistakes. Intellectual challenges also provide opportunities to develop good study habits, resiliency from surviving the inevitable mistakes, and genuine confidence that comes from knowing you can handle hard problems.
—Keith Devlin, Professor of Mathematics, Stanford University
Several of our Math Enrichment parents have asked why IMACS wasn’t mentioned in The Atlantic’s recent article entitled "The Math Revolution". Well, we’re the first to admit that we’re not sexy! To journalists, that is. Or rather what we do isn’t sexy, especially when compared to the panache of producing math competition winners. But we believe in what we do, which is more than just math. IMACS prepares children to be better thinkers for life. And after twenty-three years of seeing our approach succeed, we wouldn’t change for all the media coverage in the world.
For readers unfamiliar with IMACS, we specialize in mathematics and computer science for bright, talented and gifted K-12 students. The core of our program is our Mathematics Enrichment curriculum for elementary school students, which is taught at 29 locations throughout the US. IMACS Math Enrichment is unique in that it is intentionally designed to be completely different from school math. One might say it’s "mathematician" math because it teaches even our youngest students about beautiful and powerful mathematical structures studied by professional mathematicians and typically not introduced until college.
Why do we take this approach instead of starting with what kids have already seen and expanding on it? (It sure would be a lot easier on us to market curriculum that parents recognize and that journalists think they can write about!) This goes back to the overarching purpose of everything we do — making better thinkers for life. We’re not just about turning good math students into even better ones, although we do that. We’re not just about providing gifted students with an outlet for their creative mathematical thinking, although we do that a lot. We’re not just about teaching problem-solving skills, although we certainly do that. IMACS is about teaching children how to think in creative, logical and flexible ways that will benefit them throughout their lives, whether within or outside the mathematical arena. We equip them so that, long after they have left our classrooms and entered into the "real world", they will be able to react intelligently and constructively to problems and situations that no one alive today has even imagined.
How do you learn to face those kinds of novel situations? Not by working on problems that look familiar. As IMACS alumnus Zachary Kaufman put it, "Graduate school at Stanford was so much easier with the logical thinking skills I learned at IMACS. While classmates tried to memorize each type of problem, I was able to strongly grasp core concepts and use them to solve any problem, even if it was different from those I had seen." Zachary is a better thinker for life. Or alumna Rachel Cuozzo: "An MIT degree says to the world that you are capable of complex problem solving and abstract thinking. IMACS gave me a jumpstart on my MIT education, as it taught me to exercise the same parts of my mind that I use now on a daily basis." Rachel is a better thinker for life. This is what IMACS is about. Unfortunately, it doesn’t always make good copy in an article about math whizzes.
Furthermore, IMACS has never been only for kids who already excel at math or families seeking a challenging math education for their gifted children. Of course, the majority of our students are very mathematically talented, and they happily attend our Math Enrichment classes or take our online math courses. But IMACS also nurtures bright students with untapped abilities whose prior negative experiences turned them away from math. Usually, they have been misled into thinking that being good at math means calculating quickly, scoring high on standardized math tests, or enjoying math competitions. For many talented students, none of those apply. IMACS is able to take those kids — who often have natural talent for math expressed in other ways, such as a love for games, puzzles and patterns — and show them (1) what it means to be genuinely good at math and (2) how incredibly good at math they truly are. IMACS makes them, too, better thinkers for life.
So if you are a current IMACS parent wondering if you’re missing out on something, or if you are a prospective parent considering IMACS, we encourage you to confirm that the math enrichment providers you are considering actually live up to their claims. Some will tell you that they go beyond what schools do. Some will tell you that they don’t do "drill and kill". Verify for yourself. Ask to observe a class and to see samples of the curriculum. Does it look familiar? Is it largely similar to what schools already teach? That will tell you a lot about whether your child’s mind is going to be expanded as much and in ways that you’re hoping for and quite possibly paying for.
Last week, The Atlantic published an excellent article questioning the trend toward requiring convoluted explanations of mathematical thinking in Common Core-aligned math classes. The authors rightly pointed out that verbal explanations are hardly the only way of determining whether a student understands a concept and that many of the brightest mathematical minds are verbally challenged.
The theory that if you cannot explain, you do not understand does logically lead to the conclusion that if you understand, you can explain. (IMACS Mathematical Logic students will recognize this as an example of contrapositive inference.) That theory, however, is completely false, yet it continues to drive the misguided practices of Common Core-aligned pedagogy to the detriment of another generation of students who, we worry, will be irreparably damaged in their understanding and appreciation of math.
Visual explanations are often a natural way to demonstrate mathematical understanding when designed thoughtfully and taught well, but even they can be gamed. There is another way, however, that is an effective measure of true understanding — demonstrating how well you can apply your knowledge to a novel situation. This approach does not call specifically for a verbal, visual or symbolic explanation, but it does require that teachers have the mathematical depth to recognize understanding when presented in a variety of explanatory modes, not just how a scoring rubric of model answers dictates.
Consider for a moment the art and science of cooking. Some people can only follow a recipe, and many have compared this to when students can only "plug 'n chug" math formulas and algorithms. Some people read cooking magazines or watch the Food Network and then impress with the right vocabulary at parties, the same way verbally skilled students will learn the right Common Core-friendly phrases to use in answering certain types of problems. Then there are the chefs, the ones who understand why certain ingredients and/or cooking methods work well together and what the fancy foodie talk actually means. What can they do with their genuine understanding?
If you've never watched the television show Chopped, take a moment. Competing against the clock and other chefs, each contestant must use everything in a basket of mystery ingredients to prepare an appetizer, entrée or dessert. When the ingredients are unveiled, it is not unusual for them to include such oddities as grasshoppers, gummy bears and leftover pizza. Talk about a novel situation!
It's hard to imagine a single traditional recipe that calls for such ingredients, or what erudite words you could utter about the culinary characteristics of a grasshopper. But leave it to the chefs on Chopped to shred the pizza crust, melt the gummy bears and use them with other ingredients to make breaded and glazed gourmet grasshoppers with a pepperoni pâté!
Mathematics is a lot like cooking. When you have a genuine understanding of mathematical concepts, you know what to do when faced with a problem that is unlike any you’ve seen before but that requires putting your knowledge together in a new way. Whether you can impress the Pulitzer Prize people as well is beside the point. Bon appétit!
The Institute for Mathematics and Computer Science (IMACS) recently released its first online algebra course, Algebra: Groups, Rings and Fields. This is the tenth course in the Elements of Mathematics: Foundations (EMF) program for talented secondary school students. Our latest self-paced offering has generated a fair amount of inquiries from parents seeking options for their mathematically advanced child. The answers to some of those questions can be found in the FAQ at EMFmath.com. IMACS responds to others, which have been consolidated by topic, in this week’s blog post.
Q: My child aced pre-algebra and is ready for algebra. Why can’t I just enroll her in the first EMF algebra course?
When people use the term “algebra,” they’re usually talking about high school algebra or what mathematicians call “elementary algebra.” By contrast, EMF teaches the kind of algebra that a mathematics major at university learns called “abstract algebra.” A student who has mastered pre-algebra is, no doubt, ready for high school algebra. However, this same student, no matter how talented, is simply not ready to jump directly into abstract algebra.
Why? Just as success in high school algebra is built on the foundation laid by elementary school math up through pre-algebra, success in abstract algebra requires a strong foundation in various mathematical structures and reasoning techniques that are rarely taught outside of a university setting. This important foundation is built up through the first nine courses of the EMF program. In fact, one might think of these early courses as constituting “pre-abstract algebra.” As such, they are an integral part of the EMF program and essential to success in the later courses.
In case you’re wondering, students who complete the EMF algebra courses will have learned all of elementary algebra and be able to solve any high school algebra problem with ease. But they will also have learned a great deal more and be well-prepared to study the high-level mathematics that is at the heart of important disciplines such as particle physics and cryptography.
Q: What if my child already took high school algebra and geometry? Is there anything left for him to learn in EMF?
As IMACS principal founder and EMF co-author, Burt Kaufman, once wrote, “It is surely a sad state of affairs that in the traditional high school curricula, the student encounters very few, if any, mathematical ideas that postdate the seventeenth century. … It would be ludicrous if an English curriculum for the high school never contemplated confronting the student with a piece of literature written after Shakespeare.”* That’s one key reason why the EMF curriculum was created—to teach modern mathematics to talented, young students who are capable of benefiting from advanced material that goes beyond the outdated math curricula used in schools.
Naturally, more experienced students will find some of the EMF material familiar, but EMF approaches these topics from a far more sophisticated standpoint. Between the new mathematical structures and techniques for reasoned argument that they will be learning, there is much for these students to gain in EMF if they are motivated to learn real mathematics as opposed to just school math.
Q: We tried other math programs for advanced kids, but they just seemed to be about going faster or preparing for competitions. That’s not working for our son who’s more of a deep thinker. I’ve heard that EMF takes a different approach. Can you explain?
First, a pair of quotes:
—Jim Simons, Mathematician and Founder of Renaissance Technologies
—Jo Boaler, Professor of Mathematics Education, Stanford University
The competition-inspired approach to math has its merits. But it’s hardly the only approach worthy of mathematically talented kids. The gifted population is filled with individuals who have exceptional talent and prefer to take their time. EMF is an ideal option for these students because the program is self-paced and encourages patience in coming to a deeper understanding of complex and beautiful ideas. At the same time, we’ve had numerous EMF students who also enjoy and excel at competition math. In fact, in situations where speed is of the essence, the non-standard mathematics to which EMF students are exposed gives them a distinct advantage over others who are seeing these ideas for the first time during a competition.

EMF algebra students will have a head start in the mathematics of particle physics.
Q: You say that EMF is “mathematician math,” and that it’s taught the way a math major at university would be taught. That’s nice, but what are the benefits for a talented child who has no interest in being a math major, let alone a mathematician?
Before your child writes off being a math major completely, especially if that choice is based on experiences with school math, consider the following:
—Keith Devlin, Professor of Mathematics, Stanford University
Whether your child decides to pursue a math major or not, there are several important skills that the EMF approach to mathematics teaches. As with all IMACS programs, EMF fosters the development of logical reasoning skills in young students. While some people believe that logic is cold and inhibits creativity (think: Star Trek’s Spock!), our experience teaching the EMF curriculum over the past 30+ years suggests otherwise. To the contrary, we have found that when IMACS students are equipped with the logic skills to construct their own well-reasoned arguments and critique those of others, the clarity of thought that this produces unleashes creative and innovative ideas that were previously unfocused or muddled.
Which brings us to how EMF encourages creative thinking. As Professor Devlin reminds us, mathematics is a creative discipline. The fact that what passes as “math” in schools is devoid of creativity should not be taken as evidence that true mathematics is indifferent to creative thinking. School math tends to take a “tell-then-drill” approach where the teacher states a rule and then students apply the rule to umpteen haphazard problem sets. By contrast, EMF uses carefully constructed exercises and interactive technology to guide students to their own “discovery” of mathematical results. To be successful in EMF, a student simply must think creatively in order to cross the bridge from keen mathematical observations to the “A-ha!” moments of intuitive understanding. And when these moments happen, the joy and pride of having climbed the intellectual mountain are profound.
Another skill that EMF promotes is abstract thinking. Imagine a world in which most jobs involve people interacting with tangible objects in the present. Perhaps you envisioned the Industrial Revolution, a time when mechanical inventions led to an explosion in manufacturing. Today, we find ourselves amidst a Knowledge Revolution wherein technological inventions mean that well-paying jobs require abstract thinking about intangible ideas such as code. This is obviously true in tech, but because tech touches every industry now, it’s also true for wide-ranging fields from medicine to music to law to film. It’s the question on many people’s minds: Are you going to design and program the robot, or will you be replaced by the robot? Whatever career your child pursues, he or she will almost certainly need to think abstractly. Abstract thinking is fundamental to the study of genuine mathematics, which is what EMF teaches.
Is EMF right for your child? IMACS created a 30-minute, online Aptitude Test to help prospective parents and students answer this question. Register to take the FREE test at EMFmath.com.
* Kaufman, Burt, Jack Fitzgerald, and Jim Harpel. MEGSSS in Action. St. Louis: CEMREL, Inc., 1981.
This month’s IMACS Blog features Shiva Oswal, one of the top performers in our Elements of Mathematics: Foundations (EMF) self-paced, online program for talented secondary school students. Shiva has been enrolled in EMF since the beginning when IMACS introduced the first course of the series in late 2012. As his mom told us, “[O]ur son is an avid user of online educational resources. I recently asked him to pick his favorite online course. He answered, ‘most definitely EMF, by a wide margin’.”
Please tell our readers a little bit about yourself and what you enjoy doing.
I’ll be turning 11 soon, and I love the EMF courses! As a history buff, I really enjoy reading about decisive battles in world history. I also like playing board games and computer games. Right now my favorite board game is Robo Rally, and my favorite computer game is Castle Empire. On the weekends, I participate in Live Action Role-Playing (LARP) events. Soccer is another activity I enjoy. My favorite position is goalie.
You’ve done some amazing things already at a young age. Tell us about the accomplishments of which you are most proud.
This year, I scored 24 out of 25 to earn a gold pin in the Mathematical Olympiads for Elementary and Middle Schools (MOEMS), and was named MOEMS “Mathlete of the Year” for my geographic region. I also invented a new board game called “Minetrap”. I am currently working on publishing my game. If you would like updates, email me at shivarasul [at] gmail [dot] com. Finally, I completed the first seven EMF courses and am almost finished with the eighth course. I am excited to start the ninth course, Number Theory soon.
How did you become interested in mathematics?
When I was in preschool, I was doing multiplication. When math in regular school became too easy, I decided to homeschool so I could work on challenging math problems and concepts.
How did you become interested in taking EMF courses?
My mom encouraged me to try the EMF courses, and I’ve been hooked ever since.
What are the things you enjoy most about EMF?
I like the way concepts are explained. The format of EMF courses, reading followed by exercises, helps check my understanding. The interactive tools such as The String Game make learning math fun. Also the point system (i.e., ability to level up based on mastery of material) makes EMF addictive.
What are some ways in which your EMF experience has had a positive effect on your academic and non-academic pursuits?
When I did my first EMF course, Operational Systems, I was very new to the field. I learned a great deal about operational systems and modular arithmetic after I completed this course. I have grown by leaps and bounds as an analytical thinker as EMF courses force me to think. The program has also helped me improve my skills at strategy games like chess.
What kinds of things do you see yourself doing in the future?
First and foremost, I would like to complete all 15 EMF courses. I hope to get to Calculus before I’m 13 years old. I am also working on becoming a professional soccer player.
Thank you, Shiva, for sharing your story and congratulations on your amazing accomplishments!
Discover why the Elements of Mathematics: Foundations program from IMACS is an excellent option for your mathematically talented middle-school-aged child.
Half Price Holiday Sale: Try the first course, Operational Systems, at 50% off the regular price when you enroll on or before December 20, 2014.
Bundled Savings: Save 25% on EMF Course Pack 9, which includes the first nine courses, when you enroll on or before December 20, 2014.
Have you seen the latest video of a young child reciting multiplication tables or the digits of pi? Or maybe you know a kid who has always gotten straight A’s. Pretty neat, but does it equate to being genuinely good at math? No. Bright students often do well in school with little or no effort. And an airtight memory facilitates excellent grades, especially when those grades depend on regurgitating information that’s already been provided. Being genuinely good at math is more about having a deep understanding of how and why things work. It also means being able to take that understanding and apply it in novel situations. This is where the ability to reason logically and abstractly separates skilled thinkers from those who only learned how to go through the motions. As IMACS graduate Zachary Kaufman put it, “Graduate school at Stanford was so much easier with the logical thinking skills I learned at IMACS. While classmates tried to memorize each type of problem, I was able to strongly grasp core concepts and use them to solve any problem, even if it was different from those I had seen.” Zachary is a skilled thinker who is genuinely good at math. Will your child be?
The new school year is now a month old. By this time, most children who attend a public K-12 school in the US will have experienced the new Common Core State Standards in Mathematics (CCSSM). On the one hand, IMACS is pleased to see that key elements of the teaching philosophy we have lived by for more than 20 years are reflected in the Common Core Standards for Mathematical Practice. For a variety of reasons, however, we maintain a healthy amount of skepticism about whether the implementation of the CCSSM will lead to meaningful, positive change in mathematics education, particularly for our most talented youth.
Common Core Was Not Designed for Gifted Kids
First, the CCSSM was not designed with exceptional kids in mind. The official Common Core Web site states plainly that:
“The Standards set grade-specific standards but do not define the intervention methods or materials necessary to support students who are well below or well above grade-level expectations.”
[Source: https://www.corestandards.org/Math]
The Web site further acknowledges that Common Core, like its predecessors, cannot adequately address the unique needs of individual learners:
“No set of grade-specific standards can fully reflect the great variety in abilities, needs, learning rates, and achievement levels of students in any given classroom.”
[Source: https://www.corestandards.org/Math]
As to what educators should do about serving the diverse needs of a student body, Common Core guidance leaves them with unresolved internal conflict, offering both:
(i) “Learning opportunities will continue to vary across schools and school systems, and educators should make every effort to meet the needs of individual students based on their current understanding.”
[Source: https://www.corestandards.org/Math/Content/introduction/how-to-read-the-grade-level-standards]
and
(ii) “The Standards should be read as allowing for the widest possible range of students to participate fully from the outset, along with appropriate accommodations to ensure maximum participaton (sic) of students with special education needs.”
[Source: https://www.corestandards.org/Math]
[Note that Common Core does not include gifted children as an example of “students with special education needs.”]
But children in the right-hand tail of the distribution do have special education needs. Whether due to a failure to understand this fact, budgetary pressure, or some other constraint, some school districts seem to be latching on to (ii) above, using the arrival of Common Core as a reason to reduce or eliminate services or accommodations for gifted students. Should this become a national trend in education policy, our country will surely suffer as the majority of gifted children who rely on public education are left without appropriate alternatives.
What About Creative Problem Solvers?
Notwithstanding the potential for improving the thinking skills of typical students, the CCSSM are simply not built to inspire or nurture the creative problem solver. The unfortunate embracing of computerized testing as a cheap means of measuring “learning” — consequently resulting in a culture of teaching to the test — has made the K-12 classroom a place to dread for many unique thinkers. The plan to continue use of computerized testing under the new standards suggests that the non-standard thinker may still be out of place in the Common Core classroom.
IMACS recently asked Gerald R. Rising, SUNY Distinguished Teaching Professor Emeritus at the University at Buffalo, how he thought the CCSSM would affect mathematics education for bright children, to which he replied, “Any imposed curriculum can have a depressing effect on special programs for gifted students.” He also shared the following anecdote about the limits of standardized testing:
“On one of the tests appeared the trivial-sounding question that went something like this: ‘A workman seeks to pass a 20-foot long board through an opening with rectangular 6-foot by 8-foot cross-section. What is the maximum width of the board that is possible?’ The answer choices were: 8 feet, 9 feet, 10 feet and 11 feet. Several of our students answered 9 feet, because the board would necessarily have some thickness that would prevent a 10-foot wide board from passing through the opening. They lost full credit for thinking that was perfectly reasonable but that did not fit the professional test constructor’s overly simplistic model.”
IMACS has been delivering courses and administering tests online to bright and creative children for over 15 years, so we know a thing or two about designing effective computerized assessments of high-level thinking skills. Let’s just say that it takes tremendous creativity, foresight, and a deep understanding of how to leverage the power of technology. If high-stakes testing is here to stay, as it appears to be, we sincerely hope that the consortia working on Common Core-aligned assessments will find ways to reward (or at least not penalize) creative problem solvers.
Inadequate Investment in Training
Common Core marks a major change in teaching philosophy for math education in the US. The intent is to move away from just teaching procedural skills by giving equal weight to conceptual understanding. Teaching math with an emphasis on thinking and understanding, however, is not something one becomes proficient in after a few hours of training, which is all that many districts have provided to their teachers.
Such a radical shift in mindset can be especially challenging for some who have taught math with a completely different focus for many, many years. This is not to say that teachers are incapable of learning to teach a new way — quite the contrary. But, as with any field undergoing fundamental change, extensive training and professional development are necessary if districts and schools want a successful implementation of Common Core. So far, the evidence suggests that they cannot or will not be making that investment.
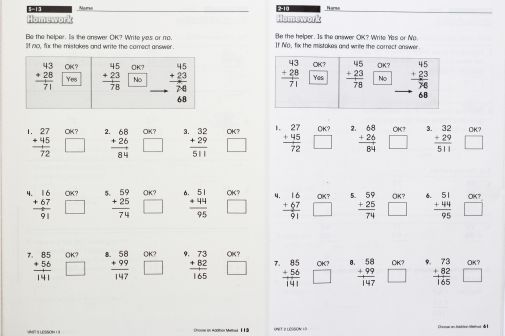
“Common Core” Textbooks In Name Only
Many of the textbooks currently on the market that say they are aligned to the Common Core standards were developed before the creation of the CCSSM. Note the example below of pages from old and new versions of a math text currently being used in California. The pages on the left were from the edition published in 2009, the year before states began adopting the CCSSM. The pages on the right are from the current edition that proclaims “Common Core” on the cover. (Click on an image to enlarge.)
Furthermore, such textbooks often only align to the specific content skills listed in the CCSSM rather than subscribing to the overall philosophy of the CCSSM. Many that claim to be aligned to the CCSSM do not include problems or tasks that involve the higher-level thinking skills that are supposed to be measured by the new Common Core standardized tests being developed.
Awareness and Advocacy Are More Important Now Than Ever
What does all this mean if you are the parent of a talented child? Probably more work for you. Just over a month ago, nearly two-thirds of respondents to a poll on education said they had never even heard of Common Core! So, if you’re thinking that someone else will speak up first, don’t count on it. Advocacy for a gifted student has never been easy given the lack of awareness and amount of misinformation about their unique educational needs. With the potential for Common Core to bring more harm than good to the education of exceptionally bright kids, it is more important now than ever to be heard.
2013 Discovery Education 3M Young Scientist Challenge.
UPDATE, May 27, 2014: IMACS student, Peyton Robertson, impressed President Barack Obama at today’s fourth annual White House Science Fair. Watch Peyton with President Obama starting around the 4:35 mark of this video.
UPDATE, October 9, 2013: Congratulations to IMACS student, Peyton Robertson, on winning the title of “America’s Top Young Scientist,” $25,000 and a trip to Costa Rica! Watch Peyton’s winning moment.
From the moment you meet IMACS student, Peyton Robertson, you can’t help feeling that he is one of those bright, young people who is going to leave his mark on the world in a big way. His creative ideas and energy seem boundless. His smile and enthusiasm are infectious. And he’s just 11 years old!
Most recently, Peyton entered the 2013 Discovery Education 3M Young Scientist Challenge and is one of only 10 finalists (and the youngest) in this prestigious national science competition for 5th through 8th graders. The Young Scientist Challenge encourages students to explore science and innovation during the pre-teen years when interest in math and science typically starts to decline.
Peyton’s project, SOS: Sandless Operational Sandbags, focuses on developing a more effective and less costly sandbag design to protect against damage from saltwater flooding. As Peyton notes in his finalist video, 80% of the $43 billion dollars worth of flood damage caused since 2005 has been from saltwater flooding. Peyton’s native Florida is at risk for hurricane-driven saltwater flooding every year. So he’s decided to do something about it by re-engineering the basic tool of flood control: the sandbag.
The design of traditional sand-filled sandbags means that they are heavy, difficult to transport and, when stacked, leave gaps through which water readily flows. Peyton’s design uses a thin, expandable polymer to keep his bags light and easy to transport. He also pre-fills his bags with enough salt so that when it dissolves, the salt content of the solution inside is higher than that of seawater, helping to keep the seawater from penetrating the bags. Finally, Peyton uses an ingenious interlocking design to minimize the gaps between the bags when they swell. When the water recedes and the bags dry out, they return to their thin, easily transported and stored form, ready for use during the next storm.
IMACS is so proud of Peyton and delighted to count him as one of our many exceptional students. When we asked Peyton’s mom, Shannon Robertson, to describe the influence that IMACS has had on his education, this is what she had to say:
“I’ll never forget our first encounter with IMACS. My son, who was three at the time, was with me at a school activities fair. He had a strong aptitude for math, so I wanted to learn more about the IMACS program. I immediately loved their focus on math and logic education. Even though we were not able to immediately enroll in the program, IMACS counseled us and gave us outstanding advice on other programs for gifted students.
Today, my son has been a part of IMACS for four years and frequently comments on how IMACS has helped him solve word problems or logic puzzles at school. IMACS fills in many of the gaps that exist in his school-based math curriculum and has given him a deeper understanding of the math that he has learned independently.
My twin girls are also in IMACS. We wanted to establish a strong foundation in logical thinking for them at an early age. After just a few months, we saw a leap forward in their math skills and critical thinking.
All three of our children look forward to their IMACS class time and dive into the supporting assignments after class. IMACS creates a uniquely fun and challenging experience for gifted students through their innovative curriculum and supportive staff. It has been an essential component of the education program for our children.”
Congratulations, Peyton! Your friends at IMACS wish you the best in the finals of the 2013 Young Scientist Challenge.
Older Posts »